Understanding Pytagoras-Finne-Side: A Comprehensive Guide
The term "Pytagoras-Finne-Side" may initially appear enigmatic, but it encapsulates concepts pivotal to mathematical applications and geometric theories. In this article, we will delve into the core principles of Pytagoras-Finne-Side, exploring its significance, mathematical background, and practical applications. Whether you are a student, educator, or math enthusiast, this guide aims to illuminate the intricacies of this fascinating topic.
What is Pytagoras-Finne-Side?
"Pytagoras-Finne-Side" combines elements from Pythagorean principles and Finnish geometric theories, offering a unique perspective on understanding side lengths in geometric shapes. While Pythagoras' Theorem deals with the relationship between the sides of a right triangle, the Finne-Side concept may refer to advanced geometric interpretations possibly rooted in Finnish mathematical studies.

pytagoras-finne-side
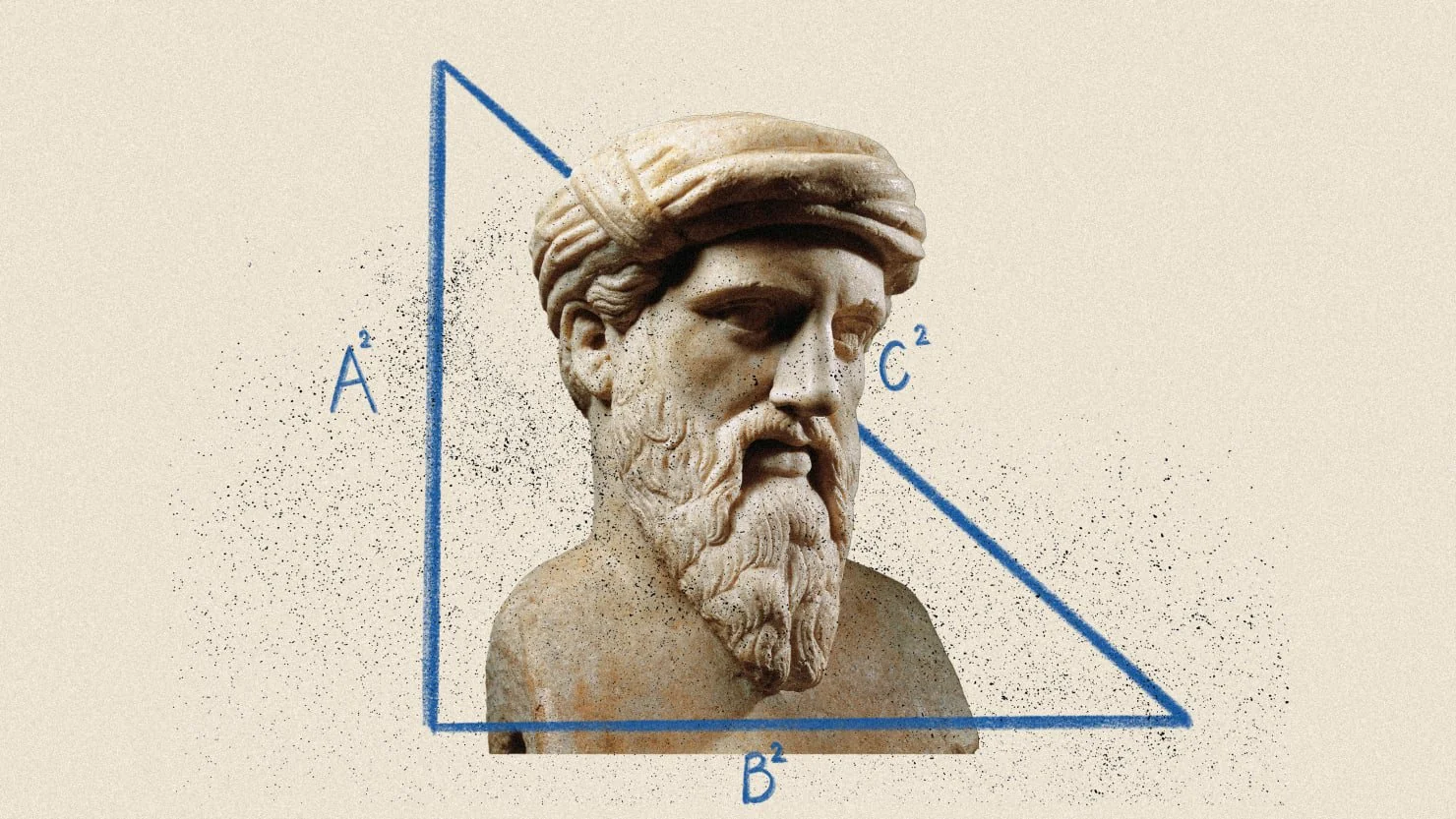
The Pythagorean Theorem
At the heart of the Pytagoras-Finne-Side concept lies the Pythagorean Theorem. This fundamental theorem in geometry states:
a2+b2=c2a^2 + b^2 = c^2a2+b2=c2
Here, aaa and bbb represent the lengths of the legs of a right triangle, while ccc denotes the length of the hypotenuse.
The Finnish Geometric Approach
The "Finne-Side" aspect likely refers to specific methodologies or geometric interpretations unique to Finnish mathematicians. Finland has a rich history of contributions to mathematical research, and combining this with Pythagorean principles could yield new insights or applications in geometry.
Key Principles of Pytagoras-Finne-Side
To understand the core principles, let’s break down the essential components:
1. Right Triangles and Hypotenuse Calculation
Using the Pythagorean Theorem, we can calculate the length of any side in a right triangle when the other two sides are known. This theorem is foundational in trigonometry and various applications in science and engineering.
2. Geometric Interpretations
The Finne-Side concept might introduce new interpretations or methodologies for solving geometric problems, potentially involving advanced Euclidean geometry or even non-Euclidean spaces.
3. Practical Applications
The combination of these principles can be applied in fields such as architecture, engineering, physics, and computer graphics, where precise calculations of angles and lengths are crucial.
pytagoras-finne-side
Applications of Pytagoras-Finne-Side
1. Architecture and Construction
Accurate calculations of side lengths and angles are vital in constructing buildings and structures. The principles of Pytagoras-Finne-Side can help architects and engineers ensure the stability and integrity of their designs.
2. Computer Graphics
In computer graphics, rendering realistic images and animations requires precise geometric calculations. Understanding these principles aids in developing algorithms for 3D modeling and simulations.
3. Education
Educators can utilize the combined principles to teach students about advanced geometric concepts, enhancing their understanding of both basic and complex mathematical theories.
Conclusion
The concept of Pytagoras-Finne-Side offers a blend of classical Pythagorean geometry with potential Finnish geometric methodologies, providing a fresh perspective on understanding side lengths and geometric interpretations. By exploring these principles, we can enhance our mathematical toolkit, applying these concepts in various practical and theoretical contexts. Whether for academic, professional, or personal enrichment, delving into the Pytagoras-Finne-Side concept opens up a world of geometric possibilities.