If (x – 5) Is a Factor of f(x), Which of the Following Must Be True? Exploring the Possibilities

In mathematics, the concept of factors plays a crucial role in understanding the properties and behavior of functions. When a polynomial function f(x) is divided by (x – 5) and yields a remainder of zero, it indicates that (x – 5) is a factor of f(x). This article aims to explore the possibilities and implications of (x – 5) being a factor of f(x) and identify the conditions that must be true in such cases.

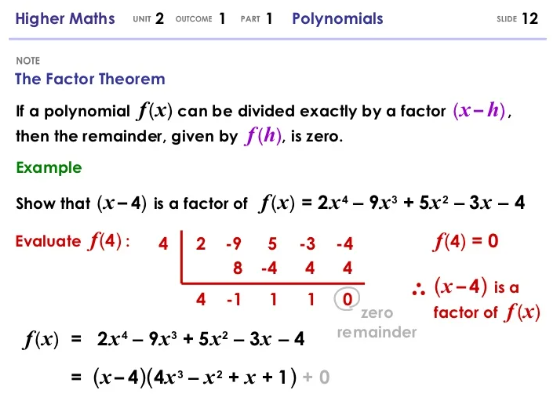
If (x – 5) is a factor of f(x), which of the following must be true?

1. Understanding Factors

Before delving into the specific scenario of (x – 5) being a factor of f(x), let's review the concept of factors.

In mathematics, factors are numbers or expressions that divide evenly into a given number or expression without leaving a remainder.

When a factor is multiplied by another factor, it yields the original number or expression.

2. (x – 5) as a Factor of f(x)

When (x – 5) is a factor of f(x), it means that f(x) can be expressed as the product of (x – 5) and another polynomial g(x). In other words, f(x) = (x – 5) * g(x), where g(x) represents the quotient obtained when f(x) is divided by (x – 5). This condition implies certain properties and relationships between f(x) and (x – 5).

3. Implications of (x – 5) Being a Factor

If (x – 5) is a factor of f(x), the following conditions must be true:

a. f(5) = 0: When (x – 5) is a factor of f(x), it means that f(5) = 0. Substituting x = 5 into the expression for f(x) results in f(5) = (5 – 5) * g(5) = 0 * g(5) = 0. Therefore, the value of f(x) at x = 5 must be zero.

b. (x – 5) is a Root of f(x): A root of a function is a value of x that makes the function equal to zero. Since (x – 5) is a factor of f(x), it implies that (x – 5) = 0 is a root of f(x). Therefore, x = 5 is a solution to the equation f(x) = 0.

c. Division Algorithm: The Division Algorithm states that any polynomial f(x) can be expressed as f(x) = (x – 5) * g(x) + r(x), where r(x) is the remainder. If (x – 5) is a factor of f(x), then the remainder r(x) must be zero.

4. Example Scenario

Let's consider an example to illustrate the implications of (x – 5) being a factor of f(x). Suppose f(x) = x^2 - 7x + 10. To determine if (x – 5) is a factor, we can use long division or synthetic division. Upon dividing f(x) by (x – 5), we obtain the quotient x - 2 and a remainder of zero. Therefore, (x – 5) is indeed a factor of f(x).

Student solving math

When (x – 5) is a factor of f(x), it indicates that f(x) can be expressed as the product of (x – 5) and another polynomial. This condition implies that f(5) = 0, (x – 5) is a root of f(x), and the remainder of the division of f(x) by (x – 5) is zero. Understanding these implications helps in analyzing the properties and behavior of functions. By exploring the possibilities and applying mathematical tools, we can determine the conditions that must be true when (x – 5) is a factor of f(x).