Tripling 3/4: Understanding the Concept and Calculation
In mathematics, understanding fractions and their operations is crucial. One common operation is multiplying a fraction by a whole number. In this article, we will explore the concept of tripling a fraction, specifically 3/4, and provide a step-by-step guide on how to calculate the result. Whether you are a student studying fractions or someone looking to refresh their math skills, this article will help you grasp the concept of tripling fractions.
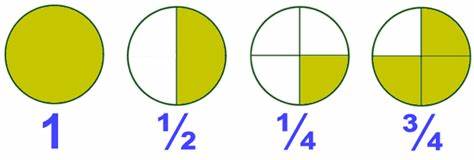
Understand concepts through circles
1. Understanding Fractions:
Before we dive into tripling a fraction, let's briefly review the basics of fractions.
A fraction represents a part of a whole or a division of a quantity into equal parts. It consists of two parts: the numerator (the number above the line) and the denominator (the number below the line).
For example, in the fraction 3/4, 3 is the numerator, and 4 is the denominator.
2. Tripling a Fraction:
Tripling a fraction simply means multiplying it by 3. In the case of 3/4, we want to find the result when this fraction is tripled. The result will be a new fraction that represents three times the original value of 3/4.
3. Step-by-Step Calculation:
To calculate the triple of 3/4, follow these steps: a. Multiply the numerator (3) by 3: 3 x 3 = 9. b. Multiply the denominator (4) by 3: 4 x 3 = 12. c. The resulting fraction is 9/12.
4. Simplifying the Result:
The fraction 9/12 can be simplified further. To simplify a fraction, find the greatest common divisor (GCD) of the numerator and denominator, and divide both by this number. In this case, the GCD of 9 and 12 is 3. Divide both numerator and denominator by 3: a. Divide 9 by 3: 9 ÷ 3 = 3. b. Divide 12 by 3: 12 ÷ 3 = 4. c. The simplified result is 3/4.
5. Interpretation:
The final result of tripling 3/4 is 3/4 itself. This means that when you triple the original value of 3/4, you still have 3/4. It indicates that 3/4 is what we call a "unit fraction" because tripling it doesn't change its value; it remains the same fraction.
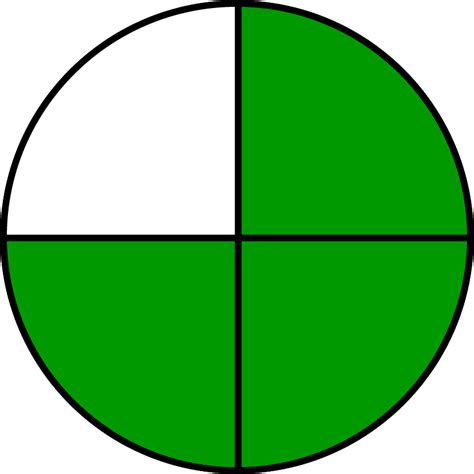
3/4 Circle
Tripling a fraction involves multiplying it by 3. In the case of 3/4, when you triple it, the result is still 3/4. Understanding fraction operations, such as tripling, is essential for building a solid foundation in mathematics. By following the step-by-step calculation and simplification process, you can confidently solve similar problems involving the multiplication of fractions. Remember, practice makes perfect, so keep exploring and practicing fractions to strengthen your math skills.